If we multiply the sides of the DIN A0 sheet, the origin of the DIN series, one by the other, as we saw last week, we obtain:
841mm x 1189mm = 1000049mm²
that is, almost exactly one square meter, as Erwin Schorr points out in his comment, with an error of just 5 hundred thousandths.
Regarding the possibility of reaching the Moon by folding paper, here is what Salva Fuster comments:
“With the thickness of the paper being approximately one tenth of a mm, by folding it 10 times we will have 210 times the initial thickness, which means having approximately 1,000 times more than the initial thickness, that is, 100 mm (0.1 m) thick. . Now, every time we fold 10 more times, the thickness will be multiplied by 1,000. Therefore, if we make 40 folds from the initial situation, we will have: 0.1 mm – 100 mm – 100 m – 100 km – 100,000 km With a couple of additional folds we would already exceed the distance to the Moon, since by making two more folds we would multiply the last of the previous values by 4. In conclusion, 42 folds is enough. Regarding the size of the sheet, taking into account that an A4 sheet of paper can be folded up to 7 times, A3 could be folded 8 times, A2 9, A1 10 and A0 11 times, that is, a sheet of 1 m². It could be folded 11 times. If we want to reach 42 folds, we will have to double it 31 times more, which would mean increasing the surface area to 2³¹ m², that is, about two billion square meters, or in other words, about 2,000 km², a size similar to the from Gipuzkoa. Now, it would surely be better to use a strip of paper and not a sheet of paper proportional to a DIN A4″.
As for the reason for the areas of the pentacle and its inner antipentacle, if you have not found it with the clues given last week you deserve to fall into the clutches of the Evil One, since the thing is as easy as realizing that the side of the pentagon regular whose diagonals form the pentacle is 1 + Φ = 2.62 times greater than the side of the inner pentagon, whose diagonals form the antipentacle; Therefore, the area of the pentacle will be 2.62² = 6.86 times greater than that of the antipentacle.
Irritating road maps
Younger people may never have had to deal with a road map, now that every cell phone and every car has access to GPS; But those of us who come from the last century have seen on more than one occasion how easy it is to unfold a map and how difficult it is to fold it properly again.
Let us consider a trivial case: a map with a single vertical fold, V1, like the one in the figure. We can only fold it in two ways: hiding the front, A, or hiding the back, R, and we can designate these folds as V1A and V1R.
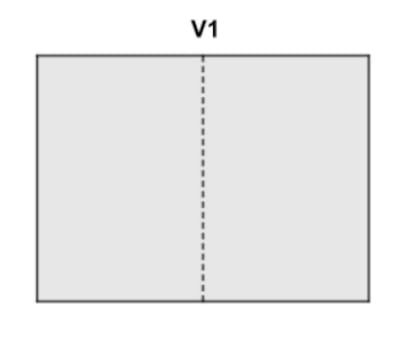
If instead of being divided into two halves, the elemental map were divided into three parts by two vertical folds (as is in fact normal in some pamphlets, not in vain called triptychs), in how many different ways could we fold it?
V1A-V2A, V1A-V2R, V1R-V2A…
Spoiler: they may seem like 8 (4 starting with V1 and 4 starting with V2), but there are actually 6 (why?). And it could also seem that 6 is the result of 3! = 3 x 2 x 1, and therefore, in the case of three vertical folds we would have 4! = 24 different bends, but it’s not like that. How many different ways can a “quatriptych” be folded?
The general problem, with n folds, which seems relatively simple, is complex and elusive, and is further complicated if, as in the case of real maps, there are both vertical and horizontal folds; In fact, a clear formula or algorithm has not yet been found to address the “irritating” (as mathematicians themselves have called it) problem of map folding.
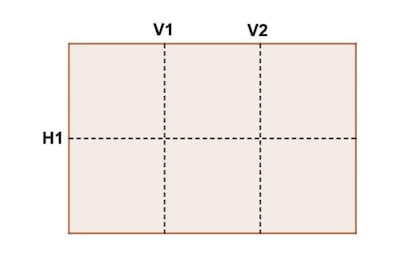
If you want to see for yourself how irritating it is, try determining how many different ways you can fold the simple map in the figure, with two vertical folds and only one horizontal fold.
You can follow SUBJECT in Facebook, x and instagramor sign up here to receive our weekly newsletter.
#art #folding #maps