The precise kiss

The coordinates of any point (P) of a circle whose center coincides with the point of intersection of the axes (O) form, together with its corresponding radius, a right triangle, in which the hypotenuse is the radius (R) and the legs are the coordinates (x, y), so, by the Pythagorean theorem, the relationship x² + y² = R2 will always hold. If the radius measures 5 units, as in the case presented last week, the requested equation will be x² + y² = 25.

Analytical geometry and its Cartesian coordinates were undoubtedly Descartes' most important contribution to mathematics, but not the only one. He often took up and gave new impetus to issues dealt with long ago, and in this sense it is worth highlighting the theorem of angular defects (which we will deal with on another occasion) and that of tangent circles.

The four tangent circles theorem

Given three circles tangent to each other two by two, we can always draw a fourth circle tangent to all three, which can be inscribed or circumscribed to them.

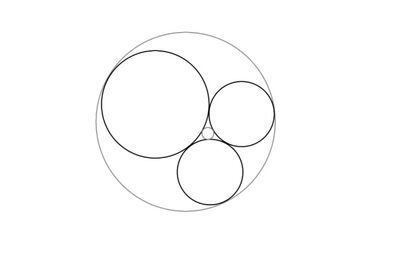
The question was addressed – we must once again quote the Great Geometer – by Apollonius of Perga, and Descartes took it up again in the mid-17th century, establishing a relationship between the respective curvatures of the four circles (remember that the curvature of a circle is the inverse of its radius, with a positive or negative sign depending on whether we consider its convex or concave part).

In 1936, the English chemist Frederick Soddy (Nobel Prize winner in chemistry in 1921) rediscovered the theorem, and published his version in the magazine Nature in the form of a humorous poem titled The Kiss Precisein which he also extends it to the case of five spheres tangent to each other (if you know English I recommend that you look for the original, easy to find on the internet; otherwise, you will have to settle for my hasty version in eneasibos):

The precise kiss

Maybe kiss in pairs

does not involve trigonometry.

It's not like that if four kiss each other

each one to the other three,

because for this they will have to be

or three in one or one in three.

If one is in three, they will receive

all three kisses from outside.

If three in one, this will be

for three kissed internally.

They manage to kiss four circles.

The smaller the more curved.

Curvature is the inverse

of the radius, the distance to the center.

And although this amazed Euclid,

no axiom is necessary.

Straight lines with zero curvature,

with minus sign concave lines,

is the addition of its squares

half square of the sum.

When spying on spherical messes,

of the osculatory watchman

It is the laborious task,

because it is the most promiscuous sphere,

and now there are more than a couple of pairs,

There are five kissing spheres.

But the signs are as before,

and by osculating each one to four,

is the square of the sum

three times the sum of squares.

After a careful reading of the poem, are you able to translate it into mathematical language – that is, to formulate Descartes' theorem – as the readers of Nature?

Before doing so, you can try to draw, with only the help of a compass (physical or mental), three circles with radii 1, 2 and 3 tangents two by two. What is the radius of the outer circle tangent to these three? And the one with the inner tangent circle?

You can follow SUBJECT in Facebook, x and instagramor sign up here to receive our weekly newsletter.

_